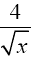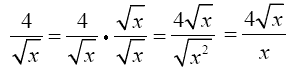Section 2: Rationalizing the Denominator
It is important that you watch the video first.
Rationalizing Denominators
The process of getting rid of the radicals in the denominator is called rationalizing the denominator. In fact, that is really what this next set of examples is about. They are really more examples of rationalizing the denominator rather than simplification examples.
Example 1
Solution.
We will make use of the fact that 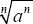
We need to determine what to multiply the denominator by so that this will show up in the denominator. Once we figure this out we will multiply the numerator and denominator by this term.
Here is the work for this part.
Remember that if we multiply the denominator by a term we must also multiply the numerator by the same term. This way we are really multiplying the term by 1, since
and so aren’t changing its value in any way.
Example 2. Rationalize the denominator.
Solution.
To rationalize the denominator, we willmake use of the fact that
(a + b)(a – b) = a² – b²
When the denominator consists of two terms with at least one of the terms involving a radical we will do the following to get rid of the radical.
So, we took the original denominator and changed the sign on the second term and multiplied the numerator and denominator by this new term. We then multiplied out the denominator to eliminate the radical.
Example 3. Rationalize the denominator.
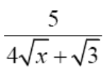
Solution.
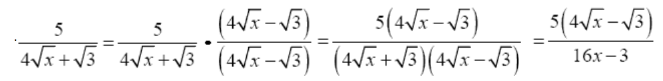
Test Your Knowledge by opening up the Test Yourself Activity.